- 1牛客网试题_牛客网题库
- 2GD32 ADC采集电压_gd32单片机adc采集电压信号
- 3Jmeter+ant+jenkins 实现接口自动化构建_ant jenkins
- 4回归预测 | MATLAB实现SABO-LSTM基于减法平均优化器优化长短期记忆神经网络的多输入单输出数据回归预测模型 （多指标，多图）_sabo算法
- 5Android 10 使用 drawable xml 设置渐变背景的坑_linear gradient requires 'angle' attribute to be a
- 6基于YOLOv8的知识蒸馏_yolov8 蒸馏
- 7打包Centos镜像，其中含有jdk,mysql,redis,ftp,nginx,rabbitmq组件_nginx jdk redis 镜像
- 8最简洁的Docker环境配置_gstream的docker环境配置
- 9Python学习从0到1 day24 第二阶段 SQL ① SQL基础语法
- 10Docker环境安装MySql详细步骤_docker安装mysql
【深度学习】DDPM，Diffusion，概率扩散去噪生成模型，原理解读_去噪扩散概率模型的数学原理
赞
踩
看过来看过去，唯有此up主，非常牛：
Video Explaination(Chinese)
1. DDPM Introduction
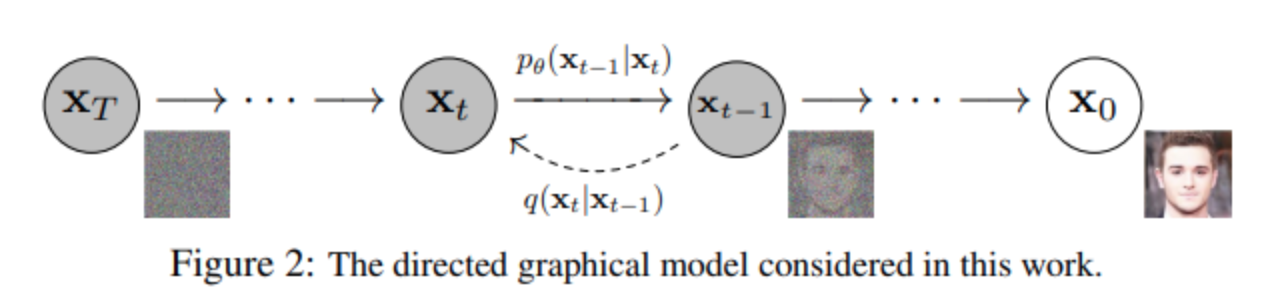
q q q - 一个固定（或预定义）的正向扩散过程，逐渐向图像添加高斯噪声，直到最终得到纯噪声。
p θ p_θ pθ - 一个学习到的反向去噪扩散过程，其中神经网络被训练以逐渐去噪图像，从纯噪声开始，直到最终得到实际图像。
正向和反向过程都由 t t t索引，发生在有限时间步数 T T T内（DDPM的作者使用 T T T=1000）。您从 t = 0 t=0 t=0开始，从数据分布中采样一个真实图像 x 0 x_0 x0，正向过程在每个时间步 t t t中从高斯分布中采样一些噪声，然后将其添加到前一个时间步的图像上。在足够大的 T T T和每个时间步添加噪声的良好安排下，通过逐渐的过程，最终在 t = T t=T t=T处得到所谓的各向同性高斯分布。
2. Forward Process q q q
这个过程是一个马尔可夫链， x t x_t xt 只依赖于 x t − 1 x_{t-1} xt−1。 q ( x t ∣ x t − 1 ) q(x_{t} | x_{t-1}) q(xt∣xt−1) 在每个时间步 t t t 按照已知的方差计划 β t β_{t} βt 添加高斯噪声。
x 0 → q ( x 1 ∣ x 0 ) x 1 → q ( x 2 ∣ x 1 ) x 2 → ⋯ → x T − 1 → q ( x t ∣ x t − 1 ) x T x_0 \overset{q(x_1 | x_0)}{\rightarrow} x_1 \overset{q(x_2 | x_1)}{\rightarrow} x_2 \rightarrow \dots \rightarrow x_{T-1} \overset{q(x_{t} | x_{t-1})}{\rightarrow} x_T x0→q(x1∣x0)x1→q(x2∣x1)x2→⋯→xT−1→q(xt∣xt−1)xT
x t = 1 − β t × x t − 1 + β t × ϵ t x_t = \sqrt{1-β_t}\times x_{t-1} + \sqrt{β_t}\times ϵ_{t} xt=1−βt ×xt−1+βt ×ϵt
- β t β_t βt is not constant at each time step t t t. In fact one defines a so-called “variance schedule”, which can be linear, quadratic, cosine, etc.
0 < β 1 < β 2 < β 3 < ⋯ < β T < 1 0 < β_1 < β_2 < β_3 < \dots < β_T < 1 0<β1<β2<β3<⋯<βT<1
- ϵ t ϵ_{t} ϵt Gaussian noise, sampled from standard normal distribution.
x t = 1 − β t × x t − 1 + β t × ϵ t x_t = \sqrt{1-β_t}\times x_{t-1} + \sqrt{β_t} \times ϵ_{t} xt=1−βt ×xt−1+βt ×ϵt
Define a t = 1 − β t a_t = 1 - β_t at=1−βt
x t = a t × x t − 1 + 1 − a t × ϵ t x_t = \sqrt{a_{t}}\times x_{t-1} + \sqrt{1-a_t} \times ϵ_{t} xt=at ×xt−1+1−at ×ϵt
2.1 Relationship between x t x_t xt and x t − 2 x_{t-2} xt−2
x t − 1 = a t − 1 × x t − 2 + 1 − a t − 1 × ϵ t − 1 x_{t-1} = \sqrt{a_{t-1}}\times x_{t-2} + \sqrt{1-a_{t-1}} \times ϵ_{t-1} xt−1=at−1 ×xt−2+1−at−1 ×ϵt−1
⇓ \Downarrow ⇓
x t = a t ( a t − 1 × x t − 2 + 1 − a t − 1 ϵ t − 1 ) + 1 − a t × ϵ t x_t = \sqrt{a_{t}} (\sqrt{a_{t-1}}\times x_{t-2} + \sqrt{1-a_{t-1}} ϵ_{t-1}) + \sqrt{1-a_t} \times ϵ_t xt=at (at−1 ×xt−2+1−at−1 ϵt−1)+1−at ×ϵt
⇓ \Downarrow ⇓
x t = a t a t − 1 × x t − 2 + a t ( 1 − a t − 1 ) ϵ t − 1 + 1 − a t × ϵ t x_t = \sqrt{a_{t}a_{t-1}}\times x_{t-2} + \sqrt{a_{t}(1-a_{t-1})} ϵ_{t-1} + \sqrt{1-a_t} \times ϵ_t xt=atat−1 ×xt−2+at(1−at−1) ϵt−1+1−at ×ϵt
Because $N(\mu_{1},\sigma_{1}^{2}) + N(\mu_{2},\sigma_{2}^{2}) = N(\mu_{1}+\mu_{2},\sigma_{1}^{2} + \sigma_{2}^{2})$x t = a t a t − 1 × x t − 2 + a t ( 1 − a t − 1 ) + 1 − a t × ϵ x_t = \sqrt{a_{t}a_{t-1}}\times x_{t-2} + \sqrt{a_{t}(1-a_{t-1}) + 1-a_t} \times ϵ xt=atat−1 ×xt−2+at(1−at−1)+1−at ×ϵ
⇓ \Downarrow ⇓
x t = a t a t − 1 × x t − 2 + 1 − a t a t − 1 × ϵ x_t = \sqrt{a_{t}a_{t-1}}\times x_{t-2} + \sqrt{1-a_{t}a_{t-1}} \times ϵ xt=atat−1 ×xt−2+1−atat−1 ×ϵ
2.2 Relationship between x t x_t xt and x t − 3 x_{t-3} xt−3
x t − 2 = a t − 2 × x t − 3 + 1 − a t − 2 × ϵ t − 2 x_{t-2} = \sqrt{a_{t-2}}\times x_{t-3} + \sqrt{1-a_{t-2}} \times ϵ_{t-2} xt−2=at−2 ×xt−3+1−at−2 ×ϵt−2
⇓ \Downarrow ⇓
x t = a t a t − 1 ( a t − 2 × x t − 3 + 1 − a t − 2 ϵ t − 2 ) + 1 − a t a t − 1 × ϵ x_t = \sqrt{a_{t}a_{t-1}}(\sqrt{a_{t-2}}\times x_{t-3} + \sqrt{1-a_{t-2}} ϵ_{t-2}) + \sqrt{1-a_{t}a_{t-1}}\times ϵ xt=atat−1 (at−2 ×xt−3+1−at−2 ϵt−2)+1−atat−1 ×ϵ
⇓ \Downarrow ⇓
x t = a t a t − 1 a t − 2 × x t − 3 + a t a t − 1 ( 1 − a t − 2 ) ϵ t − 2 + 1 − a t a t − 1 × ϵ x_t = \sqrt{a_{t}a_{t-1}a_{t-2}}\times x_{t-3} + \sqrt{a_{t}a_{t-1}(1-a_{t-2})} ϵ_{t-2} + \sqrt{1-a_{t}a_{t-1}}\times ϵ xt=atat−1at−2 ×xt−3+atat−1(1−at−2) ϵt−2+1−atat−1 ×ϵ
⇓ \Downarrow ⇓
x t = a t a t − 1 a t − 2 × x t − 3 + a t a t − 1 − a t a t − 1 a t − 2 ϵ t − 2 + 1 − a t a t − 1 × ϵ x_t = \sqrt{a_{t}a_{t-1}a_{t-2}}\times x_{t-3} + \sqrt{a_{t}a_{t-1}-a_{t}a_{t-1}a_{t-2}} ϵ_{t-2} + \sqrt{1-a_{t}a_{t-1}}\times ϵ xt=atat−1at−2 ×xt−3+atat−1−atat−1at−2 ϵt−2+1−atat−1 ×ϵ
⇓ \Downarrow ⇓
x t = a t a t − 1 a t − 2 × x t − 3 + ( a t a t − 1 − a t a t − 1 a t − 2 ) + 1 − a t a t − 1 × ϵ x_t = \sqrt{a_{t}a_{t-1}a_{t-2}}\times x_{t-3} + \sqrt{(a_{t}a_{t-1}-a_{t}a_{t-1}a_{t-2}) + 1-a_{t}a_{t-1}} \times ϵ xt=atat−1at−2 ×xt−3+(atat−1−atat−1at−2)+1−atat−1 ×ϵ
⇓ \Downarrow ⇓
x t = a t a t − 1 a t − 2 × x t − 3 + 1 − a t a t − 1 a t − 2 × ϵ x_t = \sqrt{a_{t}a_{t-1}a_{t-2}}\times x_{t-3} + \sqrt{1-a_{t}a_{t-1}a_{t-2}} \times ϵ xt=atat−1at−2 ×xt−3+1−atat−1at−2 ×ϵ
2.3 Relationship between x t x_t xt and x 0 x_0 x0
- x t = a t a t − 1 × x t − 2 + 1 − a t a t − 1 × ϵ x_t = \sqrt{a_{t}a_{t-1}}\times x_{t-2} + \sqrt{1-a_{t}a_{t-1}}\times ϵ xt=atat−1 ×xt−2+1−atat−1 ×ϵ
- x t = a t a t − 1 a t − 2 × x t − 3 + 1 − a t a t − 1 a t − 2 × ϵ x_t = \sqrt{a_{t}a_{t-1}a_{t-2}}\times x_{t-3} + \sqrt{1-a_{t}a_{t-1}a_{t-2}}\times ϵ xt=atat−1at−2 ×xt−3+1−atat−1at−2 ×ϵ
- x t = a t a t − 1 a t − 2 a t − 3 . . . a t − ( k − 2 ) a t − ( k − 1 ) × x t − k + 1 − a t a t − 1 a t − 2 a t − 3 . . . a t − ( k − 2 ) a t − ( k − 1 ) × ϵ x_t = \sqrt{a_{t}a_{t-1}a_{t-2}a_{t-3}...a_{t-(k-2)}a_{t-(k-1)}}\times x_{t-k} + \sqrt{1-a_{t}a_{t-1}a_{t-2}a_{t-3}...a_{t-(k-2)}a_{t-(k-1)}}\times ϵ xt=atat−1at−2at−3...at−(k−2)at−(k−1) ×xt−k+1−atat−1at−2at−3...at−(k−2)at−(k−1) ×ϵ
- x t = a t a t − 1 a t − 2 a t − 3 . . . a 2 a 1 × x 0 + 1 − a t a t − 1 a t − 2 a t − 3 . . . a 2 a 1 × ϵ x_t = \sqrt{a_{t}a_{t-1}a_{t-2}a_{t-3}...a_{2}a_{1}}\times x_{0} + \sqrt{1-a_{t}a_{t-1}a_{t-2}a_{t-3}...a_{2}a_{1}}\times ϵ xt=atat−1at−2at−3...a2a1 ×x0+1−atat−1at−2at−3...a2a1 ×ϵ
a ˉ t : = a t a t − 1 a t − 2 a t − 3 . . . a 2 a 1 \bar{a}_{t} := a_{t}a_{t-1}a_{t-2}a_{t-3}...a_{2}a_{1} aˉt:=atat−1at−2at−3...a2a1
x t = a ˉ t × x 0 + 1 − a ˉ t × ϵ , ϵ ∼ N ( 0 , I ) x_{t} = \sqrt{\bar{a}_t}\times x_0+ \sqrt{1-\bar{a}_t}\times ϵ , ϵ \sim N(0,I) xt=aˉt ×x0+1−aˉt ×ϵ,ϵ∼N(0,I)
⇓ \Downarrow ⇓
q ( x t ∣ x 0 ) = 1 2 π 1 − a ˉ t e ( − 1 2 ( x t − a ˉ t x 0 ) 2 1 − a ˉ t ) q(x_{t}|x_{0}) = \frac{1}{\sqrt{2\pi } \sqrt{1-\bar{a}_{t}}} e^{\left ( -\frac{1}{2}\frac{(x_{t}-\sqrt{\bar{a}_{t}}x_0)^2}{1-\bar{a}_{t}} \right ) } q(xt∣x0)=2π 1−aˉt 1e(−211−aˉt(xt−aˉt x0)2)
3.Reverse Process p p p
Because P ( A ∣ B ) = P ( B ∣ A ) P ( A ) P ( B ) P(A|B) = \frac{ P(B|A)P(A) }{ P(B) } P(A∣B)=P(B)P(B∣A)P(A)
p ( x t − 1 ∣ x t , x 0 ) = q ( x t ∣ x t − 1 , x 0 ) × q ( x t − 1 ∣ x 0 ) q ( x t ∣ x 0 ) p(x_{t-1}|x_{t},x_{0}) = \frac{ q(x_{t}|x_{t-1},x_{0})\times q(x_{t-1}|x_0)}{q(x_{t}|x_0)} p(xt−1∣xt,x0)=q(xt∣x0)q(xt∣xt−1,x0)×q(xt−1∣x0)
| | ~ | $N(\sqrt{a_t}x_{t-1}, 1-a_{t})$ |
| | ~ | $N( \sqrt{\bar{a}_{t-1}}x_0, 1-\bar{a}_{t-1})$ |
| | ~ | $N( \sqrt{\bar{a}_{t}}x_0, 1-\bar{a}_{t})$ |
q ( x t ∣ x t − 1 , x 0 ) = 1 2 π 1 − a t e ( − 1 2 ( x t − a t x t − 1 ) 2 1 − a t ) q(x_{t}|x_{t-1},x_{0}) = \frac{1}{\sqrt{2\pi } \sqrt{1-a_{t}}} e^{\left ( -\frac{1}{2}\frac{(x_{t}-\sqrt{a_t}x_{t-1})^2}{1-a_{t}} \right ) } q(xt∣xt−1,x0)=2π 1−at 1e(−211−at(xt−at xt−1)2)
q ( x t − 1 ∣ x 0 ) = 1 2 π 1 − a ˉ t − 1 e ( − 1 2 ( x t − 1 − a ˉ t − 1 x 0 ) 2 1 − a ˉ t − 1 ) q(x_{t-1}|x_{0}) = \frac{1}{\sqrt{2\pi } \sqrt{1-\bar{a}_{t-1}}} e^{\left ( -\frac{1}{2}\frac{(x_{t-1}-\sqrt{\bar{a}_{t-1}}x_0)^2}{1-\bar{a}_{t-1}} \right ) } q(xt−1∣x0)=2π 1−aˉt−1 1e(−211−aˉt−1(xt−1−aˉt−1 x0)2)
q ( x t ∣ x 0 ) = 1 2 π 1 − a ˉ t e ( − 1 2 ( x t − a ˉ t x 0 ) 2 1 − a ˉ t ) q(x_{t}|x_{0}) = \frac{1}{\sqrt{2\pi } \sqrt{1-\bar{a}_{t}}} e^{\left ( -\frac{1}{2}\frac{(x_{t}-\sqrt{\bar{a}_{t}}x_0)^2}{1-\bar{a}_{t}} \right ) } q(xt∣x0)=2π 1−aˉt 1e(−211−aˉt(xt−aˉt x0)2)
q ( x t ∣ x t − 1 , x 0 ) × q ( x t − 1 ∣ x 0 ) q ( x t ∣ x 0 ) = [ 1 2 π 1 − a t e ( − 1 2 ( x t − a t x t − 1 ) 2 1 − a t ) ] ∗ [ 1 2 π 1 − a ˉ t − 1 e ( − 1 2 ( x t − 1 − a ˉ t − 1 x 0 ) 2 1 − a ˉ t − 1 ) ] ÷ [ 1 2 π 1 − a ˉ t e ( − 1 2 ( x t − a ˉ t x 0 ) 2 1 − a ˉ t ) ] \frac{ q(x_{t}|x_{t-1},x_{0})\times q(x_{t-1}|x_0)}{q(x_{t}|x_0)} = \left [ \frac{1}{\sqrt{2\pi} \sqrt{1-a_{t}}} e^{\left ( -\frac{1}{2}\frac{(x_{t}-\sqrt{a_t}x_{t-1})^2}{1-a_{t}} \right ) } \right ] * \left [ \frac{1}{\sqrt{2\pi} \sqrt{1-\bar{a}_{t-1}}} e^{\left ( -\frac{1}{2}\frac{(x_{t-1}-\sqrt{\bar{a}_{t-1}}x_0)^2}{1-\bar{a}_{t-1}} \right ) } \right ] \div \left [ \frac{1}{\sqrt{2\pi} \sqrt{1-\bar{a}_{t}}} e^{\left ( -\frac{1}{2}\frac{(x_{t}-\sqrt{\bar{a}_{t}}x_0)^2}{1-\bar{a}_{t}} \right ) } \right ] q(xt∣x0)q(xt∣xt−1,x0)×q(xt−1∣x0)=[2π 1−at 1e(−211−at(xt−at xt−1)2)]∗[2π 1−aˉt−1 1e(−211−aˉt−1(xt−1−aˉt−1 x0)2)]÷[2π 1−aˉt 1e(−211−aˉt(xt−aˉt x0)2)]
⇓ \Downarrow ⇓
2 π 1 − a ˉ t 2 π 1 − a t 2 π 1 − a ˉ t − 1 e [ − 1 2 ( ( x t − a t x t − 1 ) 2 1 − a t + ( x t − 1 − a ˉ t − 1 x 0 ) 2 1 − a ˉ t − 1 − ( x t − a ˉ t x 0 ) 2 1 − a ˉ t ) ] \frac{\sqrt{2\pi} \sqrt{1-\bar{a}_{t}}}{\sqrt{2\pi} \sqrt{1-a_{t}} \sqrt{2\pi} \sqrt{1-\bar{a}_{t-1}} } e^{\left [ -\frac{1}{2} \left ( \frac{(x_{t}-\sqrt{a_t}x_{t-1})^2}{1-a_{t}} + \frac{(x_{t-1}-\sqrt{\bar{a}_{t-1}}x_0)^2}{1-\bar{a}_{t-1}} - \frac{(x_{t}-\sqrt{\bar{a}_{t}}x_0)^2}{1-\bar{a}_{t}} \right ) \right ] } 2π 1−at 2π 1−aˉt−1 2π 1−aˉt e[−21(1−at(xt−at xt−1)2+1−aˉt−1(xt−1−aˉt−1 x0)2−1−aˉt(xt−aˉt x0)2)]
⇓ \Downarrow ⇓
1 2 π ( 1 − a t 1 − a ˉ t − 1 1 − a ˉ t ) e x p [ − 1 2 ( ( x t − a t x t − 1 ) 2 1 − a t + ( x t − 1 − a ˉ t − 1 x 0 ) 2 1 − a ˉ t − 1 − ( x t − a ˉ t x 0 ) 2 1 − a ˉ t ) ] \frac{1}{\sqrt{2\pi} \left ( \frac{ \sqrt{1-a_t} \sqrt{1-\bar{a}_{t-1}} } {\sqrt{1-\bar{a}_{t}}} \right ) } exp{\left [ -\frac{1}{2} \left ( \frac{(x_{t}-\sqrt{a_t}x_{t-1})^2}{1-a_t} + \frac{(x_{t-1}-\sqrt{\bar{a}_{t-1}}x_0)^2}{1-\bar{a}_{t-1}} - \frac{(x_{t}-\sqrt{\bar{a}_{t}}x_0)^2}{1-\bar{a}_{t}} \right ) \right ] } 2π (1−aˉt 1−at 1−aˉt−1 )1exp[−21(1−at(xt−at xt−1)2+1−aˉt−1(xt−1−aˉt−1 x0)2−1−aˉt(xt−aˉt x0)2)]
⇓ \Downarrow ⇓
1 2 π ( 1 − a t 1 − a ˉ t − 1 1 − a ˉ t ) e x p [ − 1 2 ( x t 2 − 2 a t x t x t − 1 + a t x t − 1 2 1 − a t + x t − 1 2 − 2 a ˉ t − 1 x 0 x t − 1 + a ˉ t − 1 x 0 2 1 − a ˉ t − 1 − ( x t − a ˉ t x 0 ) 2 1 − a ˉ t ) ] \frac{1}{\sqrt{2\pi} \left ( \frac{ \sqrt{1-a_t} \sqrt{1-\bar{a}_{t-1}} } {\sqrt{1-\bar{a}_{t}}} \right ) } exp \left[ -\frac{1}{2} \left ( \frac{ x_{t}^2-2\sqrt{a_t}x_{t}x_{t-1}+{a_t}x_{t-1}^2 }{1-a_t} + \frac{ x_{t-1}^2-2\sqrt{\bar{a}_{t-1}}x_0x_{t-1}+\bar{a}_{t-1}x_0^2 }{1-\bar{a}_{t-1}} - \frac{(x_{t}-\sqrt{\bar{a}_{t}}x_0)^2}{1-\bar{a}_{t}} \right) \right] 2π (1−aˉt 1−at 1−aˉt−1 )1exp[−21(1−atxt2−2at xtxt−1+atxt−12+1−aˉt−1xt−12−2aˉt−1 x0xt−1+aˉt−1x02−1−aˉt(xt−aˉt x0)2)]
⇓ \Downarrow ⇓
1 2 π ( 1 − a t 1 − a ˉ t − 1 1 − a ˉ t ) e x p [ − 1 2 ( x t − 1 − ( a t ( 1 − a ˉ t − 1 ) 1 − a ˉ t x t + a ˉ t − 1 ( 1 − a t ) 1 − a ˉ t x 0 ) ) 2 ( 1 − a t 1 − a ˉ t − 1 1 − a ˉ t ) 2 ] \frac{1}{\sqrt{2\pi} \left ( {\color{Red} \frac{ \sqrt{1-a_t} \sqrt{1-\bar{a}_{t-1}} } {\sqrt{1-\bar{a}_{t}}}} \right ) } exp \left[ -\frac{1}{2} \frac{ \left( x_{t-1} - \left( {\color{Purple} \frac{\sqrt{a_t}(1-\bar{a}_{t-1})}{1-\bar{a}_t}x_t + \frac{\sqrt{\bar{a}_{t-1}}(1-a_t)}{1-\bar{a}_t}x_0} \right) \right) ^2 } { \left( {\color{Red} \frac{ \sqrt{1-a_t} \sqrt{1-\bar{a}_{t-1}} } {\sqrt{1-\bar{a}_{t}}}} \right)^2 } \right] 2π (1−aˉt 1−at 1−aˉt−1 )1exp −21(1−aˉt 1−at 1−aˉt−1 )2(xt−1−(1−aˉtat (1−aˉt−1)xt+1−aˉtaˉt−1 (1−at)x0))2
⇓ \Downarrow ⇓
p ( x t − 1 ∣ x t ) ∼ N ( a t ( 1 − a ˉ t − 1 ) 1 − a ˉ t x t + a ˉ t − 1 ( 1 − a t ) 1 − a ˉ t x 0 , ( 1 − a t 1 − a ˉ t − 1 1 − a ˉ t ) 2 ) p(x_{t-1}|x_{t}) \sim N\left( {\color{Purple} \frac{\sqrt{a_t}(1-\bar{a}_{t-1})}{1-\bar{a}_t}x_t + \frac{\sqrt{\bar{a}_{t-1}}(1-a_t)}{1-\bar{a}_t}x_0} , \left( {\color{Red} \frac{ \sqrt{1-a_t} \sqrt{1-\bar{a}_{t-1}} } {\sqrt{1-\bar{a}_{t}}}} \right)^2 \right) p(xt−1∣xt)∼N(1−aˉtat (1−aˉt−1)xt+1−aˉtaˉt−1 (1−at)x0,(1−aˉt 1−at 1−aˉt−1 )2)
Because x t = a ˉ t × x 0 + 1 − a ˉ t × ϵ x_{t} = \sqrt{\bar{a}_t}\times x_0+ \sqrt{1-\bar{a}_t}\times ϵ xt=aˉt ×x0+1−aˉt ×ϵ, x 0 = x t − 1 − a ˉ t × ϵ a ˉ t x_0 = \frac{x_t - \sqrt{1-\bar{a}_t}\times ϵ}{\sqrt{\bar{a}_t}} x0=aˉt xt−1−aˉt ×ϵ. Substitute x 0 x_0 x0 with this formula.
p ( x t − 1 ∣ x t ) ∼ N ( a t ( 1 − a ˉ t − 1 ) 1 − a ˉ t x t + a ˉ t − 1 ( 1 − a t ) 1 − a ˉ t × x t − 1 − a ˉ t × ϵ a ˉ t , β t ( 1 − a ˉ t − 1 ) 1 − a ˉ t ) p(x_{t-1}|x_{t}) \sim N\left( {\color{Purple} \frac{\sqrt{a_t}(1-\bar{a}_{t-1})}{1-\bar{a}_t}x_t + \frac{\sqrt{\bar{a}_{t-1}}(1-a_t)}{1-\bar{a}_t}\times \frac{x_t - \sqrt{1-\bar{a}_t}\times ϵ}{\sqrt{\bar{a}_t}} } , {\color{Red} \frac{ \beta_{t} (1-\bar{a}_{t-1}) } { 1-\bar{a}_{t}}} \right) p(xt−1∣xt)∼N(1−aˉtat (1−aˉt−1)xt+1−aˉtaˉt−1 (1−at)×aˉt xt−1−aˉt ×ϵ,1−aˉtβt(1−aˉt−1))
