【数据结构和算法】认识队列，并实现循环队列_1)设计和实现一个队列数据结构,且采用循环队列实现,采用少用一个元素的方式进行存

上接前文，我们学习了栈的相关知识内容，接下来，来认识一个与栈类似的，另一种特殊的线性表，队列，本文目的是了解并认识队列这一概念，并实现循环队列

目录

一、认识队列

1.队列的概念

2.队列的实现

二、实现循环队列

1.结构体格式以及初始化

2.队尾插入元素（入队）

3.队头删除数据（出队）

4.打印

5.清空队列、返回队头元素、返回队列元素个数

三、完整代码实现

总结

---

一、认识队列

1.队列的概念

队列：只允许在一端进行插入数据操作，在另一端进行删除数据操作的特殊的线性表，队列具有先进先出的特性，在队尾插入数据，称为入队，在队头删除数据，称为出队。

如图所示：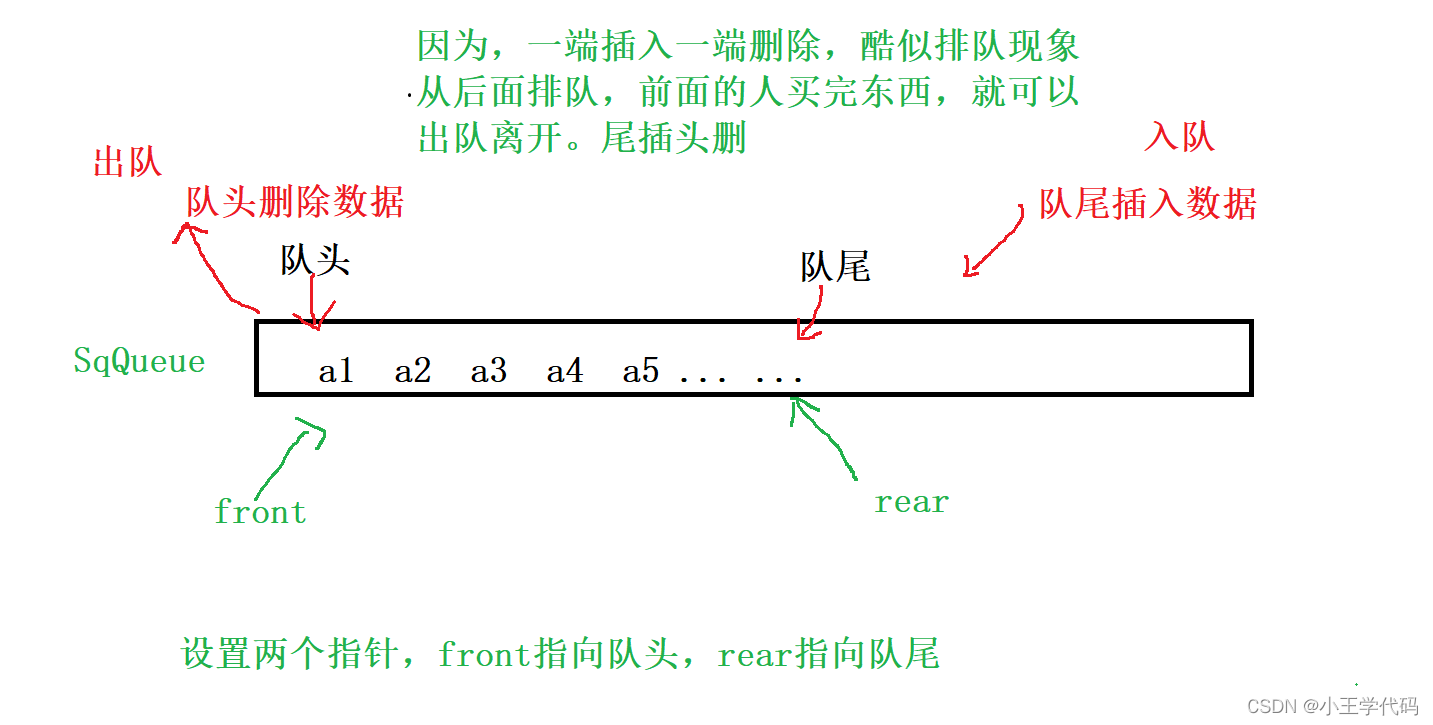

2.队列的实现

队列可以由两种方式来实现，分别可以由顺序表，和链表来实现，队列又分为循环队列，非循环队列。

区分：

1.非循环队列时，使用链表的结构实现更优一些，因为如果使用数组的结构，出队列在数组头上出数据，效率会低一点。

2.循环队列的时候，使用数组更加方便简洁，唯一缺点就是循环队列用数组的时候，只能是静态的数组，动态不能构成循环队列，当然可以使用循环链表

二、实现循环队列

我们使用数组的结构来实现循环队列

1.结构体格式以及初始化

代码如下：

#define MAXSIZE 11//表示的是数组的元素个数，多一个1，是为了留给rear空间的
typedef struct SqQueue {
	int data[MAXSIZE];
	int front;//队头
	int rear;//队尾   front 和 rear 就是指针（类似）
}SqQueue;
 
//初始化队列
void InitQueue(SqQueue* ps) {
	//初始化队列只需要队头队尾都为0   表示下标起始位置
	ps->front = ps->rear = 0;
}

实际上，我们使用的是静态数组，也只有这样我们才能实现循环队列，如果是动态数组，就无法实现循环效果，而且定义的MAXSIZE大小为11，有一个空间是不被存放数值的，作为一个标志。

2.队尾插入元素（入队）

最重要的一点是理解 

(rear+1)%MAXSIZE==front            这是判断队列是否为满队列的标志

如图所示：

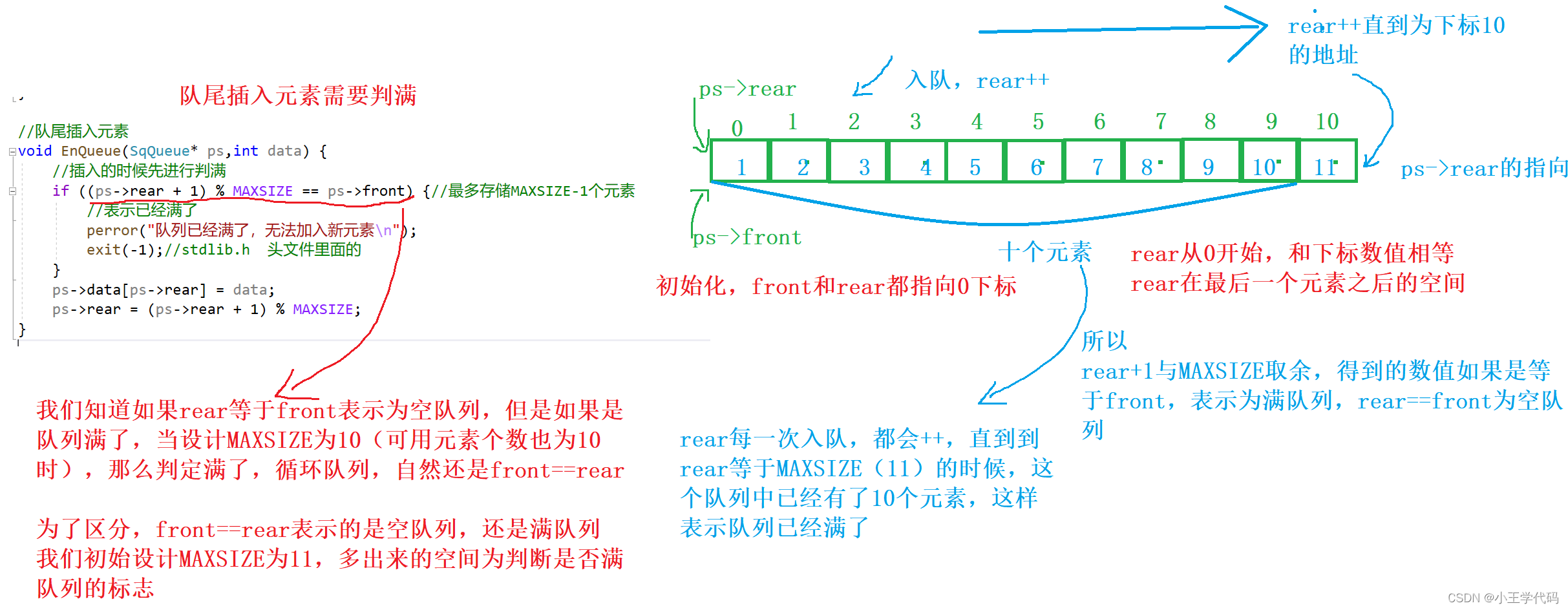
 代码如下：

//队尾插入元素
void EnQueue(SqQueue* ps,int data) {
	//插入的时候先进行判满
	if ((ps->rear + 1) % MAXSIZE == ps->front) {//最多存储MAXSIZE-1个元素
		//表示已经满了
		perror("队列已经满了，无法加入新元素\n");
		exit(-1);//stdlib.h  头文件里面的
	}
	ps->data[ps->rear] = data;
	ps->rear = (ps->rear + 1) % MAXSIZE;
}

3.队头删除数据（出队）

如图所示：

代码如下：

//队头出队
//只需要移动指针位置即可
void OutQueue(SqQueue* ps, int* data) {
	//出队先判别是否为空
	if (ps->rear == ps->front) {
		perror("为空队列，无法出队\n");
		exit(-1);
	}
	*data = ps->data[ps->front];
	ps->front = (ps->front + 1) % MAXSIZE;
	//不需要整体移动只要将队头指针后移就可以
}

4.打印

如图所示：

代码如下：

//遍历打印元素
void PrintQueue(SqQueue* ps) {
	int i = 0;
	printf("Queue->");
	while ((i + ps->front)%MAXSIZE != ps->rear) {
		//从front的队头位置开始，i从0开始 ++一直到等于rear结束
		printf("%d->", ps->data[i + ps->front]);
		i = (i + 1) % MAXSIZE;
	}
	printf("NULL\n");
}

5.清空队列、返回队头元素、返回队列元素个数

代码如下：

//将队列清空
 
void ClearQueue(SqQueue* ps) {
	ps->front = ps->rear = 0;
}
 
//返回队头元素
void GetHead(SqQueue* ps, int* data) {
	if (ps->front == ps->rear) {
		perror("为空队列\n");
		exit(-1);
	}
	*data = ps->data[ps->front];
}
 
//返回队列中的元素个数
 
int CountSqQueue(SqQueue* ps) {
	return (ps->rear - ps->front + MAXSIZE) % MAXSIZE;
}

三、完整代码实现

#define _CRT_SECURE_NO_WARNINGS
#include<stdio.h>
#include<malloc.h>
#include<assert.h>
#include<stdlib.h>
//构建的是循环队列，是使用数组的形式，完成的循环队列
 
#define MAXSIZE 11//表示的是数组的元素个数，多一个1，是为了留给rear空间的
typedef struct SqQueue {
	int data[MAXSIZE];
	int front;//队头
	int rear;//队尾   front 和 rear 就是指针（类似）
}SqQueue;
 
//初始化队列
void InitQueue(SqQueue* ps) {
	//初始化队列只需要队头队尾都为0   表示下标起始位置
	ps->front = ps->rear = 0;
}
 
//将队列清空
 
void ClearQueue(SqQueue* ps) {
	ps->front = ps->rear = 0;
}
 
//队尾插入元素
void EnQueue(SqQueue* ps,int data) {
	//插入的时候先进行判满
	if ((ps->rear + 1) % MAXSIZE == ps->front) {//最多存储MAXSIZE-1个元素
		//表示已经满了
		perror("队列已经满了，无法加入新元素\n");
		exit(-1);//stdlib.h  头文件里面的
	}
	ps->data[ps->rear] = data;
	ps->rear = (ps->rear + 1) % MAXSIZE;
}
 
//队头出队
//只需要移动指针位置即可
void OutQueue(SqQueue* ps, int* data) {
	//出队先判别是否为空
	if (ps->rear == ps->front) {
		perror("为空队列，无法出队\n");
		exit(-1);
	}
	*data = ps->data[ps->front];
	ps->front = (ps->front + 1) % MAXSIZE;
	//不需要整体移动只要将队头指针后移就可以
}
//遍历打印元素
void PrintQueue(SqQueue* ps) {
	int i = 0;
	printf("Queue->");
	while ((i + ps->front)%MAXSIZE != ps->rear) {
		//从front的队头位置开始，i从0开始 ++一直到等于rear结束
		printf("%d->", ps->data[i + ps->front]);
		i = (i + 1) % MAXSIZE;
	}
	printf("NULL\n");
}
//返回队头元素
void GetHead(SqQueue* ps, int* data) {
	if (ps->front == ps->rear) {
		perror("为空队列\n");
		exit(-1);
	}
	*data = ps->data[ps->front];
}
 
//返回队列中的元素个数
 
int CountSqQueue(SqQueue* ps) {
	return (ps->rear - ps->front + MAXSIZE) % MAXSIZE;
}
 
int main() {
	SqQueue Q;
	InitQueue(&Q);
	EnQueue(&Q, 1);
	EnQueue(&Q, 2);
	EnQueue(&Q, 3);
	EnQueue(&Q, 4);
	EnQueue(&Q, 5);
	PrintQueue(&Q);
	int a = 0;
	OutQueue(&Q, &a);
	PrintQueue(&Q);
	EnQueue(&Q, 1);
	EnQueue(&Q, 2);
	//EnQueue(&Q, 3);
	EnQueue(&Q, 4);
	EnQueue(&Q, 4);
	EnQueue(&Q, 5);
	EnQueue(&Q, 5);
	PrintQueue(&Q);
}
 

总结

本文了解认识队列这一新的概念，我们也通过数组的结构实现循环队列，这一种方式实现循环队列是比较简单好理解的，主要的是理解MAXSIZE为什么要多一个空间，例如设置为11，还有判空，判满，操作。这是最为重要的。

判空：rear==front

判满：(rear+1)%MAXSIZE==front

得到队列元素个数：(rear-front+MAXSIZE)%MAXSIZE

front、rear、i得到下一个位置的方法：

例如：rear=(rear+1)%MAXSIZE